I went off the basis of the 414m/s muzzle velocity from this video using a .8 bore musket with a .69 ball. The same size as the Brown Bess. However it isn't a 13 gram ball. That's for the Pistol. The Musketball is 32 grams. It's listed in the thread.Which is why bullets don't throw people backwards. Momentum is not at all the same thing as force, nor does it matter at all to the damage of a bullet, except that you might derive velocity from it, which can be a useful number.
We're back to the claim that flintlock pistols had half the velocity of the rifle, I see. I went back, after our last toss on this, and looked up where this entered the thread. The only provenance for this is that you said a friend messaged you and told you this fact. No source, no cite. It doesn't align with the actually sourced and cited data in the thread, which show a velocity of just below 400m/s, empirically tested. And, before the claim of "modern powder" shows up again, the flintlock muskets had about the same velocity you're claiming from whatever source you have, so modern powder cannot both replicate the muskets AND be the cause of supercharged pistols in the same study with the same methodology. I mean, they use half the charge in the pistol (they list all the pertinent data). So, no, half the velocity is a non-starter.
However, if we're looking at that 200 grain musket ball from the flintlock musket (which seems light, given the Brown Bess was over 500 grains), then the kinetic energy is 2,229 kgm^2/s^2 (I'm not sure where you get the above, are you using a different weight than 0.013kg? As I said, this looks very light, but I'm trying to stick to your numbers). To give a reference, the .44 Magnum pistol cited in your article has a kinetic energy of 1147J at the muzzle. The flintlock pistol listed in @Doug McCrae's article is 1071J. Seems we're absolutely in the danger zone with both!
Wrong units for force, and the force of the flintlock musket about is dramatically higher. The actual acceleration value isn't the same as the velocity, because it has to reach that velocity down an approx 1 meter barrel in about 4 milliseconds. Your previous calculations for bullet force are off by about 3 orders of magnitude. Acceleration is around 103,000 m/s^2, not 414. So it's not 0.013kg*414, it's 0.013kg*103,000, or 1,339N of force, in an area about 2/3 of an inch across (actually, this force will be transferred along the path -- it's not an inelastic collision so all the force isn't immediately applied).
Swords are nasty, but your evaluation of bullets is consistently very far off the mark.
I hope I've provided enough clear points, and addressed the previously cited counter-points, sufficiently well to avoid being accused of bad-faith and being blocked, again.
Which not only includes a healthy discussion of the differences between modern gunpowders but also the cavitation created by that impact. Skip forward to 9:50 and you can see nice visual charts showing the actual measurements of the temporary cavity, the purpose of using the Ballistic Gelatin in this case.
The whole "300m/s" thing comes from Flamestrike posting speeds of Musket Balls ranging from 120m/s up to 370m/s from a Wikipedia article. Y'know. Finding the Average since the musket we'd be talking about would be about 200-300 years older than the Brown Bess.
As to the pistol velocity being lower, it's based on the Wheellock Pistol video by the same person.
Skip ahead to about 18:26 for a discussion of the paper cartridge's muzzle velocity and joules. Which were lower than I got from using the speed of the flintlock pistol in the kinetic energy calculator linked previously in the thread. Same one I used to get the 2793J for the Musket at 414m/s and .032kg weight.
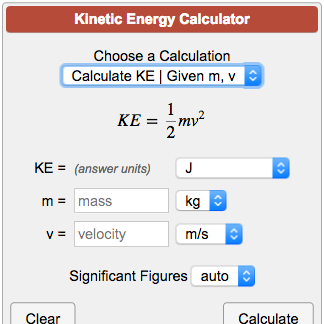
Kinetic Energy Calculator
Calculate any variable in the kinetic energy equation. Kinetic energy is equal to half the mass multiplied by velocity squared: KE = 1/2 * mv^2. Physics calculators online.
Though if you want Newtons you're gonna need this one:
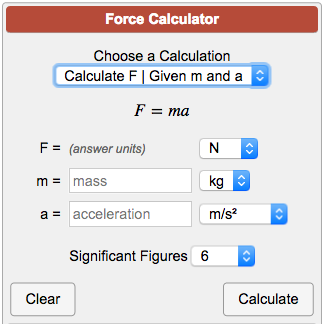
Force Calculator F = ma
Calculate the unknown variable in the equation for force, where force equals mass multiplied by acceleration. Free online physics calculators.
Using this Acceleration Calculator: Acceleration Calculator | Definition | Formula
I input 0m/s, 414m/s, and 0.024 seconds as the time interval (rounding off) for a total of 172,500m/s squared for acceleration. Toss that in the Force Calculator for the 0.032kg weight for a total of 5520N.
Just for the average muzzle velocity of muskets I went ahead and did it again with 300m/s and a time of 0.0033. Wound up with an acceleration of 90909m/s squared and a Force of 2909N.
Just for the sake of completeness, let's knock out the Wheellock while we're at it. With a Muzzle Velocity of 271m/s and a barrel length of 0.4 meters (16 inches). Because it's less than a meter I divided 1 second by the speed, then multiplied the result by 0.4 to get a time interval of 0.0014 seconds. Toss that in the acceleration calculator and we get 193,571m/s squared. Pop that into the Force Calculator with a .013kg ball and... 2516N
Somehow that feels wrong but I'm not sure where I messed up, or if I messed up. Help?
Now for the Sword. A bit wilder. But here's the source: http://swordstem.com/2018/08/22/how-fast-do-swords-move-try-1/
They provide a chart image I can't embed on these forums that shows the sword's strike time taking about 0.145 seconds. So 0 to 21m/s in 0.145 seconds into the acceleration gives us: 144m/s squared.
And then toss that into the Newtons Calculator with a 1.5kg longsword and: 217N
Yup! That is significantly tiny by comparison to the Force of the musketball. Thanks for correcting me on this! Weird that the Momentum would swing the other way.
However... The video at the start of this, with the 414m/s muzzle velocity .69 caliber bullet shows the temporary cavity as being 3 inches wide at it's largest point. Which Emergency Room Doctors say isn't a significant wounding factor. That it takes a 4 inch to 10 inch temporary cavity to provide a significant wounding factor. Something that a .44 Magnum or a modern Rifle with a tumbling and/or yawing bullet can provide.
Except in cases where an organ is struck by that temporary cavity that is particularly sensitive like the brain or liver, obviously. 3 inches is more than enough to push the no more birthday button, there, unless you're very lucky.
Last edited:
