The Mechanical Impact of -5/+10

[WhosDaDungeonMaster]

Actually, the point this thread shows, if you solve for X in at each Y in the OP, you determine the average damage at which it is more beneficial to use the -5/+10 than to not use it.

For example, at Y = 11, the formula result is 2.5 - 0.25X. If we solve for X, X = 10. If your average damage is greater than 10, you are better off NOT risking the -5 for the additional +10. If your average damage is 10, it is a break-even proposition. If your average damage is less than 10, you are better off trying the -5 penalty to increase damage by 10.



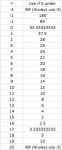
Looking at the table above, if average damage (X) is below the number listed, use the -5/+10. In the long run, you will be doing more damage on average. If you do more damage than the listed number for the Y needed to hit, don't bother as you are better off without it.

 

[WhosDaDungeonMaster]

Personally, I think the most interesting discussion around the -5/+10 mechanic is whether it should be a base rule. Is martial damage for great weapon users and archers too high, and the -5/+10 mechanic should be dropped entirely? Or would making it base rule simply allow sword and board users and two weapon fighters to do the right amount of damage?

Interesting idea. What if any character could always accept a -X penalty to attack rolls to add +2X to damage (up to the -5 limit)?

 

[Yunru]

Interesting idea. What if any character could always accept a -X penalty to attack rolls to add +2X to damage (up to the -5 limit)?

I think each on would have to be charted individually, as the crit/fumble system messes it up algebraically.

 

[Yunru]

The first post doesn't really have a topic to derail, though. The numbers are pretty well known at this point.
Personally, I think the most interesting discussion around the -5/+10 mechanic is whether it should be a base rule. Is martial damage for great weapon users and archers too high, and the -5/+10 mechanic should be dropped entirely? Or would making it base rule simply allow sword and board users and two weapon fighters to do the right amount of damage?

I can't see it being an issue as a general rule, and it would help martial characters keep up with magical.

 

[TwoSix]

I think each on would have to be charted individually, as the crit/fumble system messes it up algebraically.

Crit would really only change the math in cases where a crit could be turned into a miss by the -X penalty; that would only be cases where the character had an expanded crit range (like a champion fighter) and the hit chance was extremely low.

 

[Yunru]

Crit would really only change the math in cases where a crit could be turned into a miss by the -X penalty; that would only be cases where the character had an expanded crit range (like a champion fighter) and the hit chance was extremely low.

Nah, it's the auto-hit part that makes it complicated. It's why either end becomes a static increase.

 

[FrogReaver]

Nah, it's the auto-hit part that makes it complicated. It's why either end becomes a static increase.

You start way to many posts out with no. [MENTION=205]TwoSix[/MENTION] was correct in this instance. When you have an expanded crit range it gets very complicated as 19's can crit but don't auto hit.

You are correct in that when crit damage boost and auto hit both occur on a 20 only that the damage increase cancels out and no one has disagreed with that.

 

[Yunru]

You start way to many posts out with no. [MENTION=205]TwoSix[/MENTION] was correct in this instance. When you have an expanded crit range it gets very complicated as 19's can crit but don't auto hit.

And you're to eager to be right

The part of TwoSix's post I was disputing was that crits only change the maths with an expanded hit range.

 

[FrogReaver]

And you're to eager to be right

The part of TwoSix's post I was disputing was that crits only change the maths with an expanded hit range.

Care to give an example of crits changing the maths without an expanded crit range? I'm curious what it could possibly be.

 

[Yunru]

Care to give an example of crits changing the maths without an expanded crit range? I'm curious what it could possibly be.

Whenever you get within the five values from the end, where one of the track's chances of hitting stops changing, but the other doesn't.

So when you'd hit on a 2, the chance is the same as if you hit on a -1 (95%), but with -5, the chances become 70% and 85%.
On the other end, if you hit on a 15 or 20, the chances are 30% and 5%, but with -5, the chances both become 5%.